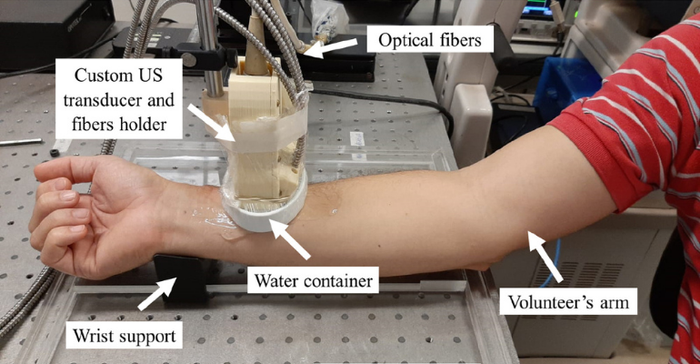
Sanguina AnemoCheck Home at-home anemia test
Packaging
Medical Test Kits Find a New HomeMedical Test Kits Find a New Home
Patients appreciate the comfort, convenience, and confidentiality of testing and treating themselves where they live.
Sign up for the QMED & MD+DI Daily newsletter.